viernes, 20 de noviembre de 2015

Cubo de las Teorias Físicas (cGh Physics)

https://en.wikipedia.org/wiki/CGh_physics

cGh physics

cGh physics refers to the mainstream attempts in physics to unify relativity, gravitation and quantum mechanics, in particular following the ideas of Matvei Petrovich Bronstein and George Gamow. The letters are the standard symbols for the speed of light (c), the gravitational constant (G), and Planck's constant (h).
If one considers these three universal constants as the basis for a 3-D coordinate system and one envisions a cube, then this pedagogical construction provides a framework referred to as the "cGh cube" or "physics cube". This cube can used for organizing major subjects within physics as occupying each of the eight corners.
The eight corners of the cGh physics cube are:

Classical mechanics (_,_,_)
Special relativity (c,_,_), Gravitation (_,G,_), Quantum mechanics (_,_,h)
General relativity (c,G,_), Quantum field theory (c,_,h), Non-relativistic quantum theory with gravity (_,G,h)
Theory of everything, or relativistic quantum gravity (c,G,h)

Other cGh subjects include Planck units, Hawking radiation and black hole thermodynamics.While there are several other physical constants, these three are given special consideration, because they can be used to define all Planck units and thus all physical quantities. The three constants are therefore used sometimes as a framework for philosophical study and as one of pedagogical patterns.

Diagram showing where quantum gravity sits in the a near-cube hierarchy of physics theories. Note that electromagnetism and quantum field theory in curved spacetime are added in as an extra and distinct items.

In popular culture

George Gamow chose "C. G. H." as the initials of his fictitious character, Mr C. G. H. Tompkins.

Matvei Petrovich Bronstein - Wikipedia, the free encyclopedia

Matvei Petrovich Bronstein (Russian: Матвей Петрович Бронштейн, December 2 [O.S. November 19] 1906, Vinnytsia — February 18, 1938) was a Soviet theoretical physicist, a pioneer of quantum gravity, author of works in astrophysics, semiconductors, quantum electrodynamics and cosmology, as well as of a number of books in popular science for children.
He introduced the cGh scheme for classifying physical theories. "After the relativistic quantum theory is created, the task will be to develop the next part of our scheme, that is to unify quantum theory (with its constant h), special relativity (with constant c), and the theory of gravitation (with its G) into a single theory."

------------------------------------------
The Physics Cube, C.J. Wainwright

Maths and Physics > The Physics Cube

The universe is goverened by three fundamental constants: The speed of light, c, Planck's constant, h, and Newton's gravitational constant, G. Advances in theoretical physics can be seen as a journey around a cube representing which of these constants we choose to include in our theories and which we choose to ignore. The ultimate goal is to include all three constants consistently in a theory of quantum gravity.

----------------

Gorelik, Gennady (1992-01-01). "Studies in the history of general relativity: First Steps of Quantum Gravity and the Planck Values". Einstein Studies (Birkhäuser Boston) 3 (1): 364–379. Retrieved 2009-05-01.

------------------

Duff, Michael; Lev B. Okun; Gabriele Veneziano (2002). "Trialogue on the number of fundamental constants". Journal of High Energy Physics (3). arXiv:physics/0110060. Bibcode:2002JHEP...03..023D. doi:10.1088/1126-6708/2002/03/023.

http://arxiv.org/abs/physics/0110060

Trialogue on the number of fundamental constants

M. J. Duff, L. B. Okun, G. Veneziano

(Submitted on 20 Oct 2001 (v1), last revised 13 Sep 2002 (this version, v3))

This paper consists of three separate articles on the number of fundamental dimensionful constants in physics. We started our debate in summer 1992 on the terrace of the famous CERN cafeteria. In the summer of 2001 we returned to the subject to find that our views still diverged and decided to explain our current positions. LBO develops the traditional approach with three constants, GV argues in favor of at most two (within superstring theory), while MJD advocates zero.

Comments:	Version appearing in JHEP; 31 pages latex
Subjects:	Classical Physics (physics.class-ph); High Energy Physics - Phenomenology (hep-ph); High Energy Physics - Theory (hep-th); General Physics (physics.gen-ph)
Journal reference:	JHEP 0203 (2002) 023
DOI:	10.1088/1126-6708/2002/03/023
Report number:	MCTP-01-45, CERN-TH/2001-277
Cite as:	arXiv:physics/0110060 [physics.class-ph]
	(or arXiv:physics/0110060v3 [physics.class-ph] for this version)

http://arxiv.org/pdf/physics/0110060v3.pdf

-----------------------------

Okun, Lev (1991-01-01). "The fundamental constants of physics". Soviet Physics Uspekhi (Sov. Phys. Usp.) 34 (9): 818–826. Bibcode:1991SvPhU..34..818O. doi:10.1070/PU1991v034n09ABEH002475. PACS 06.20.Jr.

--------------------------------------------

http://xxx.lanl.gov/abs/hep-ph/0112339

[hep-ph/0112339] Cube or hypercube of natural units
http://xxx.lanl.gov/abs/hep-ph/0112339
Cube or hypercube of natural units

L. B. Okun

(Submitted on 27 Dec 2001)

Max Planck introduced four natural units: h, c, G, k. Only the first three of them retained their status, representing the so called cube of theories, after the theory of relativity and quantum mechanics were created and became the pillars of physics. This short note is a little pebble on the tombstone of Michael Samuilovich Marinov.

Comments:	7 pages, to be published in ``Multiple facets of quantization and supersymmetry'', Michael Marinov Memorial Volume, Eds. M. Olshanetsky and A. Vainshtein, World Scientific, 2002
Subjects:	High Energy Physics - Phenomenology (hep-ph)
Cite as:	arXiv:hep-ph/0112339
	(or arXiv:hep-ph/0112339v1 for this version)

---------------------------------------------------------------------------------

LUZ Y GRAVEDAD. Año Internacional de la Luz 2015

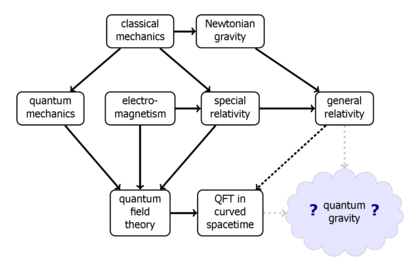
Como parte de las celebraciones mundiales de los 100 años de la Teoría de la Relatividad General de Einstein y el Año Internacional de la Luz 2015:

Ciclo de conferencias sobre la Teoría de la Relatividad General por Frederic Schuller, impartidas en la Escuela Internacional de Invierno sobre Gravedad y Luz de la universidad de Linz (Austria).
Nos dan una introducción de los fundamentos matemáticos y físicos de la teoría en 24 conferencias autónomas. Las conferencias desarrollan la teoría de los primeros principios y apuntan a un público que va desde estudiantes universitarios ambiciosos de comenzar a estudiantes de doctorado en matemáticas y física.

International Winter School On Gravity And Light 2015

Gravity and Light International Winter School 2015
FEBRUARY 14-28, 2015
AT JOHANNES KEPLER UNIVERSITY, LINZ/AUSTRIA
A full immersion course from the basics to the research frontier
Aim
In order to understand physics, one must understand the structure of spacetime.
This is because both, our most advanced theory of gravity and the description of matter — general relativity and the standard model of elementary particle physics — employ the spacetime structure in a pivotal way.
Required background of students
Background knowledge minimally required of students is what one normally would have acquired after the second year of university courses in physics and/or mathematics.
The course aims at graduate students (roughly year 4 to 5) at universities but will be accessible to advanced undergraduates and still very rewarding to beginning postgraduate students.
Philosophy of the School
A group of dedicated lecturers and distiguished experts in the field
will explain the entire mathematical and physical foundations required for a state-of-the-art command of spacetime concepts,
and thus take you on a seamless journey from your current knowledge to contemporary research highlights.

Lecturers

Frederic P. Schuller
Institute for Quantum Gravity, Erlangen

since 2012	Interim Associate Professor and Chair, Institute for Quantum Gravity, Friedrich-Alexander University Erlangen-Nuremberg, Germany
2008—2012	Researcher, Max Planck Institute for Gravitational Physics, Germany
2006—2008	Professor of Mathematical Physics, UNAM, Mexico
2005	Visting Research Fellow, The Mathematical Institute, University of Oxford, UK
2004—2006	Research Fellow, Perimeter Institute for Theoretical Physics, Canada
2004	PhD, Department of Applied Mathematics and Theoretical Physics, University of Cambridge, UK

Bernard F. Schutz
Director emiritus, Max Planck Institute for Gravitational Physics

since 1995	Director, Max Planck Institute for Gravitational Physics, Germany
since 1985	Professor of Physics and Astronomy, Cardiff University, Wales, UK Details
1976—1985	Reader in Applied Mathematics and Astronomy, Cardiff University, Wales, UK
1974—1976	Lecturer in Applied Mathematics and Astronomy, Cardiff University, Wales, UK
1973—1974	Instructor in Physics, Yale University, New Haven, CT, USA
1972—1973	Postdoctoral research assistant at Yale University, New Haven, CT, USA
1971—1972	National Science Foundation Postdoctoral Fellow at Cambridge University, Cambridge, England (Institute of Astronomy and Department of Applied Mathematics and Theoretical Physics).

Bernard F. Schutz - Wikipedia, the free encyclopedia
Bernard F. Schutz. From Wikipedia, the free encyclopedia

Bernard F. Schutz (born 11 August 1946, Paterson, New Jersey) is an American physicist. His research is on Einstein's theory of general relativity, more concretely on the physics of gravitational waves. He is one of the directors and head of the astrophysics group at the Max Planck Institute for Gravitational Physics in Potsdam, Germany. He is principal investigator in charge of data analysis for the GEO600 collaboration (which, in turn, is part of the LIGO Scientific Collaboration, the largest concerted effort to directly detect gravitational waves). Schutz is also a member of the science team coordinating the planning and development for the space-borne gravitational wave detector LISA (Laser Interferometer Space Antenna), and he was instrumental in the foundation of the electronic, open access review journal Living Reviews in Relativity.

Bibliography

Schutz, Bernard F. (1980), Geometrical methods of mathematical physics, Cambridge University Press, ISBN 0-521-29887-3

In recent years the methods of modern differential geometry have become of considerable importance in theoretical physics and have found application in relativity and cosmology, high-energy physics and field theory, thermodynamics, fluid dynamics and mechanics. This textbook provides an introduction to these methods - in particular Lie derivatives, Lie groups and differential forms - and covers their extensive applications to theoretical physics. The reader is assumed to have some familiarity with advanced calculus, linear algebra and a little elementary operator theory. The advanced physics undergraduate should therefore find the presentation quite accessible. This account will prove valuable for those with backgrounds in physics and applied mathematics who desire an introduction to the subject. Having studied the book, the reader will be able to comprehend research papers that use this mathematics and follow more advanced pure-mathematical expositions.
Common terms and phrases

affine connection arbitrary axial basis vectors calculus called canonical form chapter commute components congruence Consider const coordinate basis coordinate system d/dX defined definition differential equations differential forms differential geometry dimension dimensional dual eigenvalues element Euclidean space example Exercise exterior derivative fact fiber bundle figure follows function geodesic gives GL(n global gradient Hamiltonian hypersurface identity integral curves invariant inverse isotropy group Killing vector fields Lie algebra Lie bracket Lie derivative Lie dragging Lie group linear combination linearly independent manifold mathematical matrix metric tensor neighborhood notation one-dimensional one-form one-parameter subgroup open set operator orthonormal p-form parallel parallel-transport parameter permutation phase space physics properties prove real numbers region rotation scalar Show simply solution spacetime sphere spherical harmonics structure submanifold subspace symmetric tangent space tangent vector tensor field theorem theory three-dimensional topology transformation two-dimensional two-form unique vanish vector space volume-form zero

Schutz, Bernard F. (1985), A first course in general relativity, Cambridge University Press,376 pages ISBN 0-521-27703-5

General relativity has become one of the central pillars of theoretical physics, with important applications in both astrophysics and high-energy particle physics, and no modern theoretical physicist's education should be regarded as complete without some study of the subject. This textbook, based on the author's own undergraduate teaching, develops general relativity and its associated mathematics from a minimum of prerequisites, leading to a physical understanding of the theory in some depth. It reinforces this understanding by making a detailed study of the theory's most important applications - neutron stars, black holes, gravitational waves, and cosmology - using the most up-to-date astronomical developments. The book is suitable for a one-year course for beginning graduate students or for undergraduates in physics who have studied special relativity, vector calculus, and electrostatics. Graduate students should be able to use the book selectively for half-year courses.

Schutz, Bernard F. (2003), Gravity from the ground up: An Introductory Guide to Gravity and General Relativit
, Cambridge University Press, ISBN 0-521-45506-5

This book invites the reader to understand our Universe, not just marvel at it. From the clock-like motions of the planets to the catastrophic collapse of a star into a black hole, gravity controls the Universe. Gravity is central to modern physics, helping to answer the deepest questions about the nature of time, the origin of the Universe and the unification of the forces of nature. Linking key experiments and observations through careful physical reasoning, the author builds the reader's insight step-by-step from simple but profound facts about gravity on Earth to the frontiers of research. Topics covered include the nature of stars and galaxies, the mysteries of dark matter and dark energy, black holes, gravitational waves, inflation and the Big Bang. Suitable for general readers and for undergraduate courses, the treatment uses only high-school level mathematics, supplemented by optional computer programs, to explain the laws of physics governing gravity.
Contents

gravity takes center stage	9
what goes up doesnt always come down	19
a triumph for Newtonian gravity	25
the real signature of gravity	39
the cosmic rollercoaster	51
CONTENTS ix	72
keeping the heat on	85
the emptiness of outer space	103

finding out what is not relative	211
Einstein climbs onto Newtons shoulders	225
fashioning the geometry of gravity	239
laboratories of strong gravity	261
gravitys oneway street	285
gravity speaks	309
bringing the Universe into focus	331
the study of everything	345

why they are black bodies	109
factories for the Universe	121
the life cycle of the stars	135
tidal forces on a huge scale	153
atoms in the Universe	163
Einstein stands on Galileos shoulders	179
logic and experiment in relativity	195

the seed from which we grew	367
the geometry of cosmology	383
cosmic questions at the frontiers of gravity	391
values of useful constants	419
Index	443
Copyright

oooo

Schutz, Bernard F. (May 31, 2009), A first course in general relativity (2 ed.), Cambridge University Press, ISBN 0-521-88705-4

References

American Men and Women of Science, Thomson Gale, 2004

External links

Astrophysics Group at the Albert Einstein Institute

Domenico Giulini
Professor for Theoretical Physics

since 2014	Chair of the Gravitation and Relativity Section of the German Physical Society
since 2013	Member of the Board of Directors, Riemann Center for Geometry and Physics, Hannover, Germany
since 2009	Professor, Institute for Theoretical Physics, University of Hannover, Germany
2007—2009	Researcher, Max Planck Institute for Theoretical Physics, Germany
1997—2007	Research and Teaching, Universities Zurich, Freiburg, Karlsruhe
1996	Habilitation and venia legendi, University of Freiburg, Germany
1990	PhD, Department of Applied Mathematics and Theoretical Physics, University of Cambridge, UK

Marcus C. Werner
Yukawa Institute Japan and Cambridge University

from 2015	Assistant Professor, Yukawa Institute for Theoretical Physics, Japan
since 2012	Adjunct Assistant Professor, Department of Mathematics, Duke University, USA
since 2011	Research Fellow Kavli Institute for the Physics and the Mathematics of the Universe, Japan
2009—2011	Visiting Assistant Professor Department of Mathematics, Duke University, USA
2005—2009	PhD University of Cambridge, UK

Valeria Pettorino
ITP Heidelberg

Junior Research Group Leader for HGSFP and ITP Cosmology Group, University of Heidelberg, Germany

Marie Curie Fellow, University of Geneva, Switzerland

Postdoctoral Fellow, SISSA Trieste, Italy

Alexander von Humboldt Fellow, University of Heidelberg, Germany

Postdoctoral Fellow, Torino, Italy

PhD, University of Naples, Italy

Schedule

First Week	Sunday	Monday	Tuesday	Wednesday	Thursday	Friday	Saturday
9-10.15am	Mathematical Revision Course	Main Lecture on Gravity and Light	Workshop	Main Lecture on Gravity and Light	Excursion: Ars Electronica Center	Workshop	Main Lecture on Gravity and Light
10.15-10.45am	Coffee Break					Coffee Break
10.45-12am	Mathematical Revision Course	Main Lecture on Gravity and Light	Workshop	Main Lecture on Gravity and Light		Workshop	Main Lecture on Gravity and Light
12-2pm	Lunch					Lunch
2-4pm	Mathematical Revision Course	Tutorial	Classic Journal Club	Tutorial		Classic Journal Club	Tutorial
4-4.30pm	Coffee break
4.30-6pm	Mathematical Revision Course	Excursion 1	Excursion 2		Satellite Lecture	Satellite Lecture
6-8pm	Dinner			Dinner
8-9pm	Opera			Evening Lecture

Second Week	Sunday	Monday	Tuesday	Wednesday	Thursday	Friday	Saturday
9-10.15am		Main Lecture on Gravity and Light	Workshop	Main Lecture on Gravity and Light	Workshop	Main Lecture on Gravity and Light	Presentation of Workshop Results
10.15-10.45am		Coffee Break
10.45-12am		Main Lecture on Gravity and Light	Workshop	Main Lecture on Gravity and Light	Workshop	Main Lecture on Gravity and Light	Presentation of Workshop Results
12-2pm	Lunch
2-4pm		Tutorial	Classic Journal Club	Tutorial	Classic Journal Club	Tutorial	Final Meeting
4-4.30pm		Coffee break
4.30-6pm		Satellite Lecture	Satellite Lecture		Satellite Lecture	Satellite Lecture
6-8pm	Dinner
8-9pm	Evening Lecture			Evening Lecture

Modules
The aim of the school is achieved by way of three well-balanced pillars:
A 15-hour online course on differential geometry (held and made available two weeks before the school)
A 15-hour lecture course (coherently introducing the theories of general relativity and light)
A 12-hour satellite lecture program by four different experts (taking you to the research frontier)
A 12-hour series of tutorials by excellent teaching assistants (to practice the basics)
A 10-hour series of workshops (where more advanced problems are solved in teams)
An 8-hour classical papers journal club (linking the modern theory to its historical and philosophical roots)
Keynote evening lectures on gravitational waves, lensing, the microwave background and quantum gravity
Tour and practical observations at the Kepler observatory in Linz
Cinema viewing of the Kepler Opera (providing a window into Kepler's life and time)

Gravity and Light International Winter School 2015 ?
http://www.gravity-and-light.org/lectures ?
http://www.gravity-and-light.org/tutorials ?

Google+

https://www.youtube.com/channel/UCUHKG3S9N_QeIE2jQXd2-VQ/about
As part of the world-wide celebrations of the 100th anniversary of Einstein's theory of general relativity and the International Year of Light 2015:
Central lecture course by Frederic P Schuller (A thorough introduction to the theory of general relativity) introducing the mathematical and physical foundations of the theory in 24 self-contained lectures at the International Winter School on Gravity and Light in Linz/Austria.
The lectures develop the theory from first principles and aim at an audience ranging from ambitious undergraduate students to beginning PhD students in mathematics and physics.
Satellite Lectures (see other videos on this channel) by Bernard F Schutz (Gravitational Waves), Domenico Giulini (Canonical Formulation of Gravity), Marcus C Werner (Gravitational Lensing) and Valeria Pettorino (Cosmic Microwave Background) expand on the topics of this central lecture course and take students to the research frontier
International Year of Light - The We-Heraeus International Winter School on Gravity and Light
A full immersion course from the basics to the research frontier
February 14, 2015 - February 28, 2015
--------------------------------------------------------------------------
International Winter School on Gravity and Light 2015 facebook

https://www.facebook.com/WinterSchoolGravityLight/videos/1511514979132992/

lllllllllllllllllllllllllllllllllllllllllllllllllllllllllllllllllllllllllllllllllllllllllllllllllllllllllllllllllllllllllllll
The WE-Heraeus International Winter School on Gravity and Light - YouTube

Listas de reproducción creadas

Evening lectures 2 videos

de
2 videos 208 vistas Se actualizó por última vez el 11/05/2015
Reproducir todos

Evening Lecture A: Gravitational Waves: Music on the Spheres (Winter School on Gravity and Light) - Duración: 48:09 de 258 vistas

https://youtu.be/udmiKlH7-AA?list=PLFeEvEPtX_0SvGlcapJIULAYIjMwWw3rZ
<iframe width="853" height="480" src="https://www.youtube.com/embed/udmiKlH7-AA?list=PLFeEvEPtX_0SvGlcapJIULAYIjMwWw3rZ" frameborder="0" allowfullscreen></iframe>

Evening Lecture B: Quantum versus Gravity (International Winter School on Gravity and Light 2015) - Duración: 1:33:13 de 357 vistas

https://youtu.be/bxzTD7usU1s?list=PLFeEvEPtX_0SvGlcapJIULAYIjMwWw3rZ

<iframe width="853" height="480" src="https://www.youtube.com/embed/bxzTD7usU1s?list=PLFeEvEPtX_0SvGlcapJIULAYIjMwWw3rZ" frameborder="0" allowfullscreen></iframe>

Series | Scienceface Video Clip Series about Science Today
------------------------------------------

Central Lecture Course 28 videos

de
28 videos 12,975 vistas Se actualizó por última vez el 29/03/

https://www.youtube.com/playlist?list=PLFeEvEPtX_0S6vxxiiNPrJbLu9aK1UVC_
<iframe width="853" height="480" src="https://www.youtube.com/embed/videoseries?list=PLFeEvEPtX_0S6vxxiiNPrJbLu9aK1UVC_" frameborder="0" allowfullscreen></iframe>

Publicado el 10/02/2015

As part of the world-wide celebrations of the 100th anniversary of Einstein's theory of general relativity and the International Year of Light 2015, the Scientific Organizing Committee makes available the central 24 lectures by Frederic P Schuller.
Central lecture course by Frederic P Schuller (A thorough introduction to the theory of general relativity) introducing the mathematical and physical foundations of the theory in 24 self-contained lectures at the International Winter School on Gravity and Light in Linz/Austria.
Titled "A thorough introduction to the theory of general relativity", the lectures introduce the mathematical and physical foundations of the theory in 24 self-contained lectures. The material is developed step by step from first principles and aims at an audience ranging from ambitious undergraduate students to beginning PhD students in mathematics and physics.
The lectures develop the theory from first principles and aim at an audience ranging from ambitious undergraduate students to beginning PhD students in mathematics and physics.
Satellite Lectures (see other videos on this channel) by Bernard F Schutz (Gravitational Waves), Domenico Giulini (Canonical Formulation of Gravity), Marcus C Werner (Gravitational Lensing) and Valeria Pettorino (Cosmic Microwave Background) expand on the topics of this central lecture course and take students to the research frontier.
Access to further material on www.gravity-and-light.org/lectures and www.gravity-and-light.org/tutorials

Lecture 1: Topology (International Winter School on Gravity and Light 2015) - YouTube
https://youtu.be/7G4SqIboeig?list=PLFeEvEPtX_0S6vxxiiNPrJbLu9aK1UVC_

<iframe width="853" height="480" src="https://www.youtube.com/embed/7G4SqIboeig?list=PLFeEvEPtX_0S6vxxiiNPrJbLu9aK1UVC_" frameborder="0" allowfullscreen></iframe>

a) The power set (P) of a set (M) is the set which contains all subsets of that set. u∈P(M) <-> u⊆M
b) A topology (O) can be defined on a set (M) as a subset of the power set

-i) a topology must contain the set (M) and the empty set. ∅,M∈O (∴{∅,M}⊆O⊆P(M))
-ii) the intersection of any two members of a topology must also be a member of the topology. (v∩u)∈O | u,v∈O
-iii) the union of any number of members of the topology must also result in a member of the topology. Ui(u)∈O | u∈O (is there any reason it needs to be an indexed set rather than simply v∪u like the previous axiom?)

c) Members of a topology are called open sets d) A set is closed if it's compliment (relative in M) is an open set e) A map (f) from set M to set N is continuous if the preimage (with respect to f) of every open set in N is an open set in M (obviously requireing a topology in both). ∀V∈O:preim(V)∈O f) If we have 2 maps (f:M->N and g:N->P) and they're both continuous, then the composition of the two is also continuous. g) A subset (S) of a set with a topology can inherit that topology by taking the intersection of the subset and every element in the topology. Os = {u∩S|u∈O} h) If you restrict a continuous map to a specific subset in the domain and inherit the topology, then the restricted map is still continuous.
Nice synopsis for such a long video eh?

Lecture 2: Topological Manifolds (International Winter School on Gravity and Light 2015) - Duración: 1:23:01 de 5,597 vistas
https://youtu.be/93f-ayezCqE?list=PLFeEvEPtX_0S6vxxiiNPrJbLu9aK1UVC_

<iframe width="853" height="480" src="https://www.youtube.com/embed/93f-ayezCqE?list=PLFeEvEPtX_0S6vxxiiNPrJbLu9aK1UVC_" frameborder="0" allowfullscreen></iframe>

Lecture 3: Multilinear Algebra (International Winter School on Gravity and Light 2015) - Duración: 1:42:36 de 4,498 vistas
https://youtu.be/mbv3T15nWq0?list=PLFeEvEPtX_0S6vxxiiNPrJbLu9aK1UVC_

<iframe width="853" height="480" src="https://www.youtube.com/embed/mbv3T15nWq0?list=PLFeEvEPtX_0S6vxxiiNPrJbLu9aK1UVC_" frameborder="0" allowfullscreen></iframe>

This is the most thorough explanation of the vector space I have ever heard (13:43). Thank you for the nice lecture.

Lecture 4: Differentiable Manifolds (International Winter School on Gravity and Light 2015) - Duración: 1:00:24 de 3,982 vistas

Lecture 5: Tangent Spaces (International Winter School on Gravity and Light 2015) - Duración: 1:36:41 de 3,835 vistas

Lecture 6: Fields (International Winter School on Gravity and Light 2015) - Duración: 1:15:10 de 2,319 vistas

Lecture 7: Connections (International Winter School on Gravity and Light 2015) - Duración: 1:43:22 de 2,095 vistas

Lecture 8: Parallel Transport & Curvature (International Winter School on Gravity and Light 2015) - Duración: 1:27:12 de 2,242 vistas

Lecture 9: Newtonian spacetime is curved! (International Winter School on Gravity and Light 2015) - Duración: 1:48:32 de 1,188 vistas

https://www.youtube.com/watch?v=IBlCu1zgD4Y
https://youtu.be/IBlCu1zgD4Y

<iframe width="853" height="480" src="https://www.youtube.com/embed/IBlCu1zgD4Y" frameborder="0" allowfullscreen></iframe>

Lecture 9: Newtonian spacetime is curved! (International Winter School on Gravity and Light 2015) - YouTube

<iframe width="853" height="480" src="https://www.youtube.com/embed/IBlCu1zgD4Y" frameborder="0" allowfullscreen></iframe>

Lecture 10: Metric Manifolds (International Winter School on Gravity and Light 2015) - Duración: 1:20:28 de 1,292 vistas

Lecture 11: Symmetry (International Winter School on Gravity and Light 2015) - Duración: 1:43:30 de 1,136 vistas

Lecture 12: Integration on manifolds (International Winter School on Gravity and Light 2015) - Duración: 1:28:15 de 1,128 vistas

Lecture 13: Spacetime (International Winter School on Gravity and Light 2015) - Duración: 1:51:16 de 1,215 vistas

Lecture 14: Matter (International Winter School on Gravity and Light 2015) - Duración: 1:00:28 de 676 vistas

https://youtu.be/65Y38aRWIXs

<iframe width="853" height="480" src="https://www.youtube.com/embed/65Y38aRWIXs" frameborder="0" allowfullscreen></iframe>

Lecture 15: Einstein Gravity (International Winter School on Gravity and Light 2015) - Duración: 1:06:09 de 820 vistas

<iframe width="853" height="480" src="https://www.youtube.com/embed/ZfewnqcBYag" frameborder="0" allowfullscreen></iframe>

Lecture 16: Optical Geometry I (International Winter School on Gravity and Light 2015) - Duración: 1:14:06 de 603 vistas

Lecture 17: Optical Geometry II (International Winter School on Gravity and Light 2015) - Duración: 1:38:59 de 348 vistas

https://youtu.be/2-nOtRESiUc

Lecture 18: Canonical Formulation of GR I (International Winter School on Gravity and Light 2015) - Duración: 1:41:15 de 547 vistas

Lecture 19: Canonical Formulation of GR II (International Winter School on Gravity and Light 2015) - Duración: 2:01:21 de 309 vistas

Lecture 20: Cosmology - The early epoch (International Winter School on Gravity and Light 2015) - Duración: 1:39:22 de 507 vistas

Lecture 21: Cosmology - The late epoch (International Winter School on Gravity and Light 2015) - Duración: 1:17:32 de 293 vistas

Lecture 22: Black Holes (International Winter School on Gravity and Light 2015) - Duración: 1:37:58 de 601 vistas

Publicado el 27/02/2015

https://youtu.be/7VJzouE9rNE

<iframe width="853" height="480" src="https://www.youtube.com/embed/7VJzouE9rNE" frameborder="0" allowfullscreen></iframe>

Lecture 23: Penrose Diagrams (International Winter School on Gravity and Light 2015) - Duración: 1:28:02 de 780 vistas

Lecture 24: Perturbation Theory I (International Winter School on Gravity and Light 2015) - Duración: 1:28:10 de 531 vistas

Lecture 25: Perturbation Theory II (International Winter School on Gravity and Light 2015) - Duración: 1:38:55 de 384 vistas

Lecture 26: How quantizable matter gravitates (International Winter School on Gravity and Light) - Duración: 1:39:13 de 668 vistas

Lecture 27: Sources of gravitational waves (International Winter School on Gravity and Light 2015) - Duración: 1:42:25 de 342 vistas

Lecture 28: How to detect gravitational waves (International Winter School on Gravity and Light) - Duración: 1:21:43 de 323 vistas

Tutorials 11 videos

Tutorials - The WE-Heraeus International Winter School on Gravity and Light

Tutorial 1: Topology (International Winter School on Gravity and Light 2015) - Duración: 32:35 de 1,103 vistas

<iframe width="853" height="480" src="https://www.youtube.com/embed/_XkhZQ-hNLs" frameborder="0" allowfullscreen></iframe>

Tutorial 2: Topological Manifolds (International Winter School on Gravity and Light 2015) - Duración: 29:37 de 433 vistas

<iframe width="853" height="480" src="https://www.youtube.com/embed/ghfEQ3u_B6g" frameborder="0" allowfullscreen></iframe>

Tutorial 3: Multilinear Algebra (International Winter School on Gravity and Light 2015) - Duración: 57:19 de 419 vistas

<iframe width="853" height="480" src="https://www.youtube.com/embed/5oeWX3NUhMA" frameborder="0" allowfullscreen></iframe>

Tutorial 4: Differentiable Manifolds (International Winter School on Gravity and Light 2015) - Duración: 35:24 de 441 vistas

Tutorial 5: Tangent Spaces (International Winter School on Gravity and Light 2015) - Duración: 1:32:26 de 533 vistas

Tutorial 11: Symmetries (International Winter School on Gravity and Light 2015) - Duración: 59:57 de 326 vistas

https://youtu.be/bYfvT-ky1lU
<iframe width="853" height="480" src="https://www.youtube.com/embed/bYfvT-ky1lU" frameborder="0" allowfullscreen></iframe>

Tutorial 12: Integration (International Winter School on Gravity and Light 2015) - Duración: 20:13 de 236 vistas

Tutorial 13: Schwarzschild Spacetime (International Winter School on Gravity and Light 2015) - Duración: 33:32 de 214 vistas
https://youtu.be/KQe2sqGIzTg
<iframe width="853" height="480" src="https://www.youtube.com/embed/KQe2sqGIzTg" frameborder="0" allowfullscreen></iframe>

Tutorial 15: Cosmology (International Winter School on Gravity and Light 2015) - Duración: 43:32 de 182 vistas

https://youtu.be/HuQ79CWcDac
<iframe width="853" height="480" src="https://www.youtube.com/embed/HuQ79CWcDac" frameborder="0" allowfullscreen></iframe>

Tutorial 16: Diagrams (International Winter School on Gravity and Light 2015) - Duración: 36:25 de 182 vistas